HCF Full Form: What is HCF?

What is HCF?

HCF Full Form: HCF, which stands for Highest Common Factor, is a fundamental concept in mathematics. It represents the largest factor that two or more numbers have in common. Typically introduced around the 4th or 5th grade, understanding HCF is crucial for simplifying fractions and solving various mathematical problems involving divisors.

Steps to Find the HCF

To find the HCF of any set of numbers, follow these steps:

1. List the Factors: Write down all the factors of each number.
2. Identify Common Factors: Look for the common factors in all the lists.
3. Select the Highest Factor: Among the common factors, the highest one is the HCF.

By following these steps, you can determine the HCF of any given numbers.

Key Concepts: Factors and Prime Factorization

What is a Factor?

A factor is a number that divides another number without leaving a remainder. For example, 2 and 4 are factors of 8 because 2 × 4 = 8.

Properties of Factors: HCF Full Form

• 1 is the smallest factor of any number.
• The number itself is always the greatest factor.
• All factors of a number are less than or equal to the number.

How to Find Factors

There are two main methods to find factors: the Factor Pair Method and the Prime Factorization Method. HCF Full Form

Factor Pair Method

Multiplication Method: To find the factors of 24 using multiplication: HCF Full Form

• 1 × 24 = 24
• 2 × 12 = 24
• 3 × 8 = 24
• 4 × 6 = 24

Thus, the factors of 24 are 1, 2, 3, 4, 6, 8, 12, and 24.

Division Method: To find the factors of 24 using division: HCF Full Form

• 24 ÷ 1 = 24
• 24 ÷ 2 = 12
• 24 ÷ 3 = 8
• 24 ÷ 4 = 6
• 24 ÷ 6 = 4
• 24 ÷ 8 = 3
• 24 ÷ 12 = 2
• 24 ÷ 24 = 1

Again, the factors of 24 are 1, 2, 3, 4, 6, 8, 12, and 24.

Prime and Composite Numbers

• Prime Numbers: A prime number is a number greater than 1 that has no factors other than 1 and itself. Examples include 2, 3, 5, 7, and 11.
• Composite Numbers: A composite number is a number greater than 1 that has more than two factors. Examples include 4, 6, 8, 9, and 12.

Prime Factorization

Prime factorization involves breaking down a number into its prime factors. For instance, the prime factorization of 24 is: 24=2×2×2×3=23×324 = 2 \times 2 \times 2 \times 3 = 2^3 \times 324=2×2×2×3=23×3 

Methods of Prime Factorization

Factor Tree Method

The Factor Tree method is a simple and visual way to break down a number into its prime factors. Let’s walk through the steps using the number 24: HCF Full Form

1. Start with the smallest prime factor of 24: 2.
2. Divide 24 by 2: This gives us 12.
3. Find the smallest prime factor of 12: 2.
4. Divide 12 by 2: This gives us 6.
5. Find the smallest prime factor of 6: 2.
6. Divide 6 by 2: This gives us 3.
7. 3 is a prime number: We stop here.

So, the prime factorization of 24 is: 24=2×2×2×3=23×324 = 2 \times 2 \times 2 \times 3 = 2^3 \times 324=2×2×2×3=23×3

Common Division Method

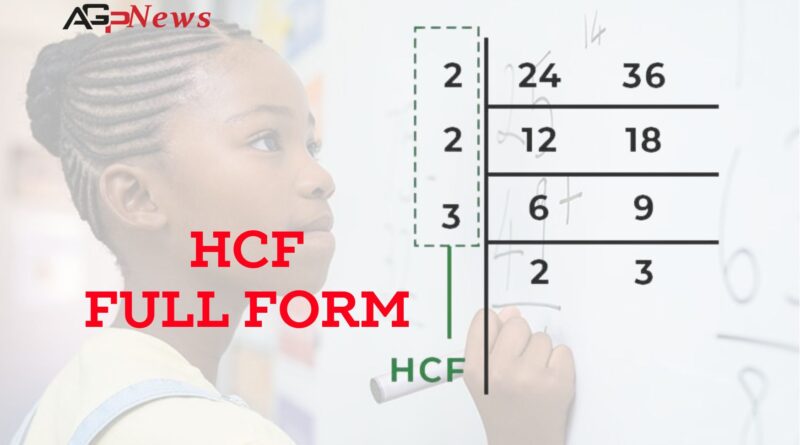
The Common Division method is another straightforward approach to prime factorization, focusing on division: HCF Full Form

1. Start by dividing 24 by the smallest prime factor, 2: This gives us 12.
2. Divide 12 by 2: This gives us 6.
3. Divide 6 by 2: This gives us 3.
4. 3 is a prime number: We stop here.

So, the prime factorization of 24 using common division is also: 24=2×2×2×3=23×324 = 2 \times 2 \times 2 \times 3 = 2^3 \times 324=2×2×2×3=23×3

Finding Common Factors

To find common factors, compare the factors of two or more numbers. Let’s use the numbers 24 and 32 as an example.

• Factors of 24: 1, 2, 3, 4, 6, 8, 12, 24
• Factors of 32: 1, 2, 4, 8, 16, 32

Common factors of 24 and 32: 1, 2, 4, 8

Finding the Highest Common Factor (HCF)

To find the HCF, identify the highest number in the list of common factors. Let’s find the HCF of 32 and 44.

• Factors of 32: 1, 2, 4, 8, 16, 32
• Factors of 44: 1, 2, 4, 11, 22, 44

Common factors of 32 and 44: 1, 2, 4

The highest common factor (HCF) of 32 and 44 is 4.

Conclusion

Understanding the HCF Full Form (Highest Common Factor) is essential for solving various mathematical problems. By learning to identify factors, perform prime factorization, and find common factors, you can easily determine the HCF of any set of numbers. This fundamental concept is not only crucial in mathematics but also in simplifying fractions and solving problems related to divisors.